Great geophysicists #10: Joseph Fourier

Joseph Fourier, the great mathematician, was born on 21 March 1768 in Auxerre, France, and died in Paris on 16 May 1830, aged 62. He's the reason I didn't get to study geophysics as an undergraduate: Fourier analysis was the first thing that I ever struggled with in mathematics.

Fourier was one of 12 children of a tailor, and had lost both parents by the age of 9. After studying under Lagrange at the École Normale Supérieure, Fourier taught at the École Polytechnique. At the age of 30, he was an invited scientist on Napoleon's Egyptian campaign, along with 55,000 other men, mostly soldiers:

Citizen, the executive directory having in the present circumstances a particular need of your talents and of your zeal has just disposed of you for the sake of public service. You should prepare yourself and be ready to depart at the first order.
Herivel, J (1975). Joseph Fourier: The Man and the Physicist, Oxford Univ. Press.

He stayed in Egypt for two years, helping found the modern era of Egyptology. He must have liked the weather because his next major work, and the one that made him famous, was Théorie analytique de la chaleur (1822), on the physics of heat. The topic was incidental though, because it was really his analytical methods that changed the world. His approach of decomposing arbitrary functions into trignometric series was novel and profoundly useful, and not just for solving the heat equation. 

Fourier as a geophysicist

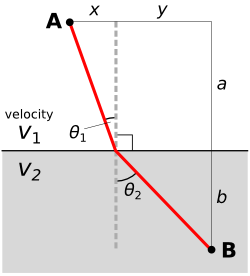
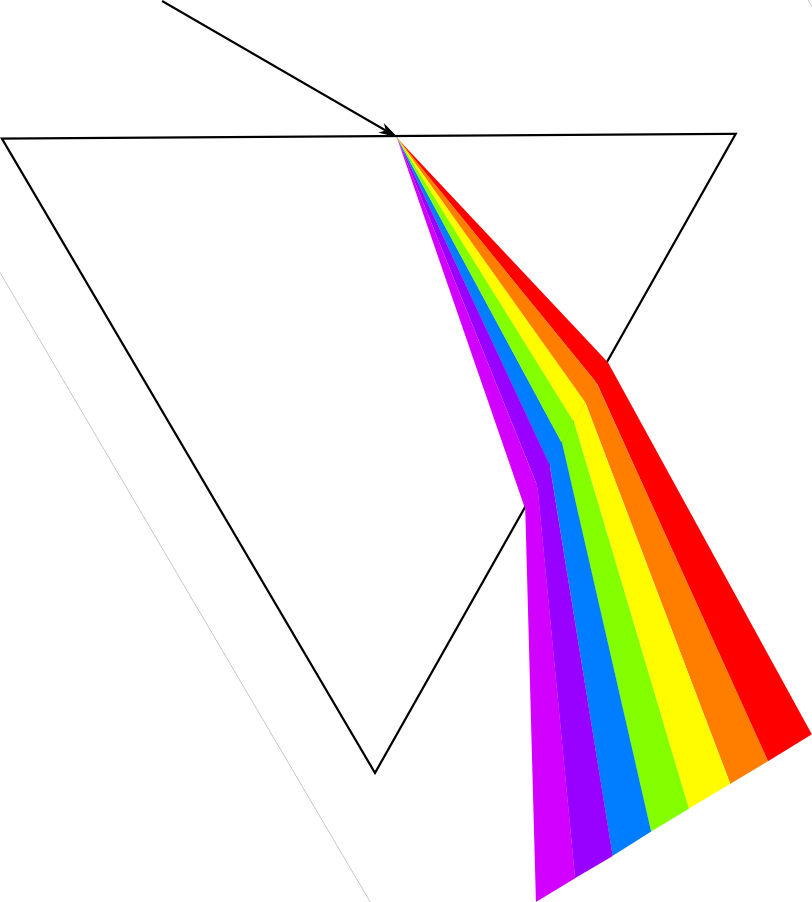
Late last year, Evan wrote about the reason Fourier's work is so important in geophysical signal processing in Hooray for Fourier! He showed how we can decompose time-based signals like seismic traces into their frequency components. And I touched the topic in K is for Wavenumber (decomposing space) and The spectrum of the spectrum (decomposing frequency itself, which is even weirder than it sounds). But this GIF (below) is almost all you need to see both the simplicity and the utility of the Fourier transform. 

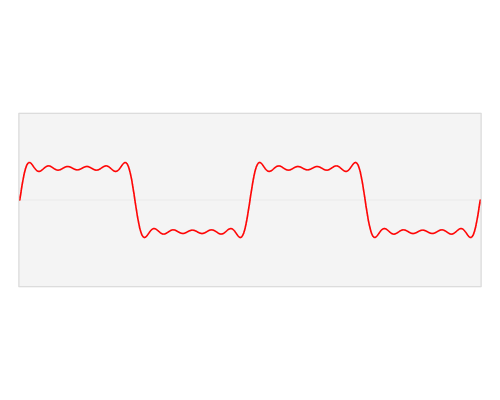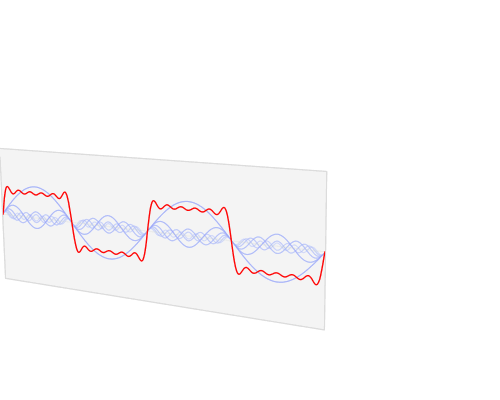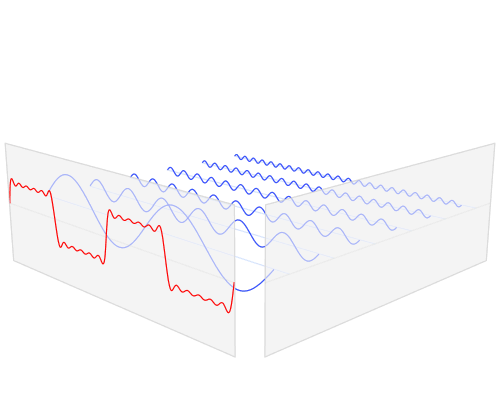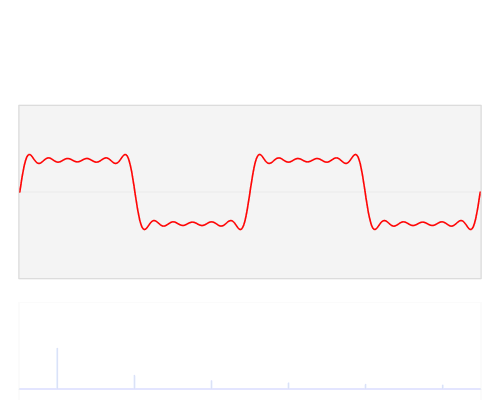In this example, we start with something approaching a square wave (red), and let's assume it's in the time domain. This wave can be approximated by summing the series of sine waves shown in blue. The amplitudes of the sine waves required are the Fourier 'coefficients'. Notice that we needed lots of time samples to represent this signal smoothly, but require only 6 Fourier coefficients to carry the same information. Mathematicians call this a 'sparse' representation. Sparsity is a handy property because we can do clever things with sparse signals. For example, we can compress them (the basis of the JPEG scheme), or interpolate them (as in CGG's REVIVE processing). Hooray for Fourier indeed.

The watercolour caricature of Fourier is by Julien-Leopold Boilly from his work Album de 73 Portraits-Charge Aquarelle’s des Membres de I’Institute (1820); it is in the public domain.

Read more about Fourier on his Wikipedia page — and listen to this excellent mini-biography by Marcus de Sautoy. And check out Mostafa Naghizadeh's chapter in 52 Things You Should Know About Geophysics. Download the chapter for free!

Great geophysicists #9: Ernst Chladni

Ernst Chladni was born in Wittenberg, eastern Germany, on 30 November 1756, and died 3 April 1827, at the age of 70, in the Prussian city of Breslau (now Wrocław, Poland). Several of his ancestors were learned theologians, but his father was a lawyer and his mother and stepmother from lawyerly families. So young Ernst did well to break away into a sound profession, ho ho, making substantial advances in acoustic physics. 

Chladni, 'the father of acoustics', conducted a large number of experiments with sound, measuring the speed of sound in various solids, and — more adventurously — in several gases too, including oxygen, nitrogen, and carbon dioxode. Interestingly, though I can find only one reference to it, he found that the speed of sound in Pinus sylvestris was 25% faster along the grain, compared to across it — is this the first observation of acoustic anisotropy? 

The experiments Chladni is known for, however, are the plates. He effectively extended the 1D explorations of Euler and Bernoulli in rods, and d'Alembert in strings, to the 2D realm. You won't find a better introduction to Chladni patterns than this wonderful blog post by Greg Gbur. Do read it — he segués nicely into quantum mechanics and optics, firmly linking Chladni with the modern era. To see the patterns forming for yourself, here's a terrific demonstration (very loud!)...

The drawings from Chladni's book Die Akustik are almost as mesmerizing as the video. Indeed, Chladni toured most of mainland Europe, demonstrating the figures live to curious Enlightenment audiences. When I look at them, I can't help wondering if there is some application for exploration geophysics — perhaps we are missing something important in the wavefield when we sample with regular acquisition grids?

References

Chladni, E, Die Akustik, Breitkopf und Härtel, Leipzig, 1830. Amazingly, this publishing company still exists.

Read more about Chladni in Wikipedia and in monoskop.org — an amazing repository of information on the arts and sciences. 

This post is part of a not-very-regular series of posts on important contributors to geophysics. It's going rather slowly — we're still in the eighteenth century. See all of them, and do make suggestions if we're missing some!

Great geophysicists #8: d'Alembert

Jean-Baptiste le Rond d'Alembert was a French mathematician, born on 16 or 17 November 1717 in Paris, and died on 29 October 1783, also in Paris. His father was an artillery officer, but his mother was much more interesting. Having been a nun, she sought papal dispensation in 1714 for a new career as a fun-loving socialite, benefiting from the new government banknote printing scheme of John Law. She left her illegitimate child on the steps of Église St Jean Le Rond de Paris, whence he was taken to an orphanage. When his father returned from duty, he arranged for the boy's care.

Perhaps d'Alembert's greatest contribution to the world was helping Denis Diderot 'change the way people think' by editing the great Encyclopédie, ou Dictionnaire raisonné des sciences, des arts et des métiers of 1751. There were many contributors, but d'Alembert was listed as co-editor on the title page (left). This book was an essential ingredient in spreading the Enlightenment across Europe, and d'Alembert was closely involved in the project for at least a decade. 

But that's not why he's in our list of great geophysicists. As I mentioned when I wrote about Euler, d'Alembert substantially progressed the understanding of waves, making his biggest breakthrough in 1747 in his work on vibrating strings. His paper was the first time the wave equation or its solution had appeared in print:

Though Euler and d'Alembert corresponded on waves and other matters, and strongly influenced each other, they eventually fell out. For example, Euler wrote to Lagrange in 1759:

d'Alembert has tried to undermine [my solution to the vibrating strings problem] by various cavils, and that for the sole reason that he did not get it himself... He thinks he can deceive the semi-learned by his eloquence. I doubt whether he is serious, unless perhaps he is thoroughly blinded by self-love. [See Morris Kline, 1972]

D'Alembert did little mathematics after 1760, as he became more involved in other academic matters. Later, ill health gradually took over. He lamented to Lagrange (evidently an Enlightenment agony aunt) in 1777, six years before his death:

What annoys me the most is the fact that geometry, which is the only occupation that truly interests me, is the one thing that I cannot do. [See Thomas Hankins, 1970]

I imagine he died feeling a little hollow about his work on waves, unaware of the future impact it would have—not just in applied geophysics, but in communication, medicine, engineering, and so on. For solving the wave equation, d'Alembert, we salute you.

References

Read more on Wikipedia and The MacTutor History of Mathematics.

D'Alembert, J-B (1747). Recherches sur la courbe que forme une corde tenduë mise en vibration. (Researches on the curve that a tense cord forms [when] set into vibration.) Histoire de l'académie royale des sciences et belles lettres de Berlin, vol. 3, pages 214–219. Read on Google Books, with its sister paper, 'Further researches...'.

Portrait is a pastel by Maurice Quentin de La Tour, 1704–88.

Great geophysicists #7: Leonhard Euler

Leonhard Euler (pronounced 'oiler') was born on 15 April 1707 in Basel, Switzerland, but spent most of his life in Berlin and St Petersburg, where he died on 18 September 1783. Has was blind from the age of 50, but took this handicap stoically—when he lost sight in his right eye at 28 he said, "Now I will have less distraction".

It's hard to list Euler's contributions to the toolbox we call seismic geophysics—he worked on so many problems in maths and physics. For example, much of the notation we use today was invented or at least popularized by him: f (x), e, i, π. He reconciled Newton's and Liebnitz's versions of calculus, making huge advances in solving difficult real-world equations. But he made some particularly relevant advances that resonate still:

• Leonardo and Galileo both worked on mechanical stress distribution in beams, but didn't have the luxuries of calculus or Hooke's law. Daniel Bernoulli and Euler developed an isotropic elastic beam theory, and eventually convinced people you could actually build things using their insights. 
• Euler's equations of fluid dynamics pre-date the more complicated (i.e. realistic) Navier–Stokes equations. Nonetheless, this work continued into vibrating strings, getting Euler (and Bernoulli) close to a general solution of the wave equation. They missed the mark, however, leaving it to Jean-Baptiste le Rond d'Alembert. 
• optics (also wave behaviour). Though many of Euler's ideas about dispersion and lenses turned out to be incorrect (e.g. Pedersen 2008, DOI 10.1162/posc.2008.16.4.392), Euler did at least progress the idea that light is a wave, helping scientists move away from Newton's corpuscular theory.

The moment of Euler's death was described by the Marquis de Condorcet in a eulogy:

He had full possession of his faculties and apparently all of his strength... after having enjoyed some calculations on his blackboard concerning the laws of ascending motion for aerostatic machines... [he] spoke of Herschel's planet and the mathematics concerning its orbit and a little while later he had his grandson come and play with him and took a few cups of tea, when all of a sudden the pipe that he was smoking slipped from his hand and he ceased to calculate and live.

"He ceased to calculate," I love that.

Great geophysicists #6: Robert Hooke

Robert Hooke was born near Freshwater on the Isle of Wight, UK, on 28 July 1635, and died on 13 March 1703 in London. At 18, he was awarded a chorister scholarship at Oxford, where he studied physics under Robert Boyle, 8 years his senior. 

Hooke's famous law tells us how things deform and, along with Newton, Hooke is thus a parent of the wave equation. The derivation starts by equating the force due to acceleration (of a vibrating particle, say), and the force due to elastic deformation:

where m is mass, x is displacement, the two dots denote the second derivative with respect to time (a.k.a. acceleration), and k is the spring constant. This powerful insight, which allows us to compute a particle's motion at a given time, was first made by d'Alembert in about 1742. It is the founding principle of seismic rock physics.

Hooke the geologist

Like most scientists of the 17th century, Hooke was no specialist. One of his best known works was Micrographia, first published in 1665. The microscope was invented in the late 1500s, but Hooke was one of the first people to meticulously document and beautifully draw his observations. His book was a smash hit by all accounts, inspiring wonder in everyone who read it (Samuel Pepys, for example). Among other things, Hooke described samples of petrified wood, forams, ammonites, and crystals of quartz in a flint nodule (left). Hooke also wrote about the chalk formations in the cliffs near his home town.

Hooke went on to help Wren rebuild London after the great fire of 1666, and achieved great respect for this work too. So esteemed is he that Newton was apparently rather jealous of him, and one historian has referred to him as 'England's Leonardo'. He never married, and lived in his Oxford college all his adult life, and is buried in Bishopsgate, London. As one of the fathers of geophysics, we salute him.

The painting of Hooke, by Rita Greer, is licensed under a Free Art License. It's a interpretation based on descriptions of him ("his chin sharp, and forehead large"); amazingly, there are no known contemporary images of him. Hear more about this.

You can read more about the relationship between Hooke's law and seismic waves in Bill Goodway's and Evan's chapters in 52 Things You Should Know About Geophysics. Download their chapters for free!

Great geophysicists #5: Huygens

Christiaan Huygens was a Dutch physicist. He was born in The Hague on 14 April 1629, and died there on 8 July 1695. It's fun to imagine these times: he was a little older than Newton (born 1643), a little younger than Fermat (1601), and about the same age as Hooke (1635). He lived in England and France and must have met these men.

It's also fun to imagine the intellectual wonder life must have held for a wealthy, educated person in these protolithic Enlightenment years. Everyone, it seems, was a polymath: Huygens made substantial contributions to probability, mechanics, astronomy, optics, and horology. He was the first to describe Saturn's rings. He invented the pendulum clock. 

Then again, he also tried to build a combustion engine that ran on gunpowder. 

Geophysicists (and most other physicists) know him for his work on wave theory, which prevailed over Newton's corpuscles—at least until quantum theory. In his Treatise on Light, Huygens described a model for light waves that predicted the effects of reflection and refraction. Interference has to wait 38 years till Fresnel. He even explained birefringence, the anisotropy that gives rise to the double-refraction in calcite.

The model that we call the Huygens–Fresnel principle consists of spherical waves emanating from every point in a light source, such as a candle's flame. The sum of these manifold wavefronts predicts the distribution of the wave everywhere and at all times in the future. It's a sort of infinitesimal calculus for waves. I bet Newton secretly wished he'd thought of it.

Great geophysicists #4: Fermat

This Friday is Pierre de Fermat's 411th birthday. The great mathematician was born on 17 August 1601 in Beaumont-de-Lomagne, France, and died on 12 January 1665 in Castres, at the age of 63. While not a geophysicist sensu stricto, Fermat made a vast number of important discoveries that we use every day, including the principle of least time, and the foundations of probability theory. 

Fermat built on Heron of Alexandria's idea that light takes the shortest path, proposing instead that light takes the path of least time. These ideas might seem equivalent, but think about anisotropic and inhomogenous media. Fermat continued by deriving Snell's law. Let's see how that works.

We start by computing the time taken along a path:

Then we differentiate with respect to space. This effectively gives us the slope of the graph of time vs distance.

We want to minimize the time taken, which happens at the minimum on the time vs distance graph. At the minimum, the derivative is zero. The result is instantly recognizable as Snell's law:

Maupertuis's generalization

The principle is a core component of the principle of least action in classical mechanics, first proposed by Pierre Louis Maupertuis (1698–1759), another Frenchman. Indeed, it was Fermat's handling of Snell's law that Maupertuis objected to: he didn't like Fermat giving preference to least time over least distance.

Maupertuis's generalization of Fermat's principle was an important step. By the application of the calculus of variations, one can derive the equations of motion for any system. These are the equations at the heart of Newton's laws and Hooke's law, which underlie all of the physics of the seismic experiment. So, you know, quite useful.

Probably very clever

It's so hard to appreciate fundamental discoveries in hindsight. Together with Blaise Pascal, he solved basic problems in practical gambling that seem quite straightforward today. For example, Antoine Gombaud, the Chevalier de Méré, asked Pascal: why is it a good idea to bet on getting a 1 in four dice rolls, but not on a double-1 in twenty-four? But at the time, when no-one had thought about analysing problems in terms of permutations and combinations before, the solutions were revolutionary. And profitable.

For setting Snell's law on a firm theoretical footing, and introducing probability into the world, we say Pierre de Fermat (pictured here) is indeed a father of geophysics.

Pair picking

Even the Lone Ranger didn't work alone all of the timeImagine that you are totally entrained in what you are doing: focused, dedicated, and productive. If you've lost track of time, you are probably feeling flow. It's an awesome experience when one person gets it, imagine the power when teams get it. Because there are so many interruptions that can cause turbulence, it can be especially difficult to establish coherent flow for the subsurface team. But if you learn how to harness and hold onto it, it's totally worth it.

Seismic interpreters can seek out flow by partnering up and practising pair picking. Having a partner in the passenger seat is not only ideal for training, but it is a superior way to get real work done. In other industries, this has become routine because it works. Software developers sometimes code in pairs, and airline pilots share control of an aircraft. When one person is in charge of the controls, the other is monitoring, reviewing, and navigating. One person for tactical jobs, one for strategic surveillance.

Here are some reasons to try pair picking:

Solve problems efficiently — If you routinely have an affiliate, you will have someone to talk to when you run into a challenging problem. Mundane or sticky workarounds become less tenuous when you have a partner. You'll adopt more sensible solutions to your fit-for-purpose hacks.

Integrate smoothly — There's a time for hand-over, and there will be times when you must call upon other people's previous work to get your job done. 'No! Don't use Top_Cretaceous_candidate_final... use Evan_K_temp_DO-NOT-USE.' Pairing with the predecessors and successors of your role will get you better-aligned.

Minimize interruptionitis — if you have to run to a meeting, or the phone rings, your partner can keep plugging away. When you return you will quickly rejoin. It is best to get into a visualization room, or some other distraction-free room with a large screen, so as to keep your attention and minimize the effect of interruptions.

Mutual accountability — build allies based on science, technology, and critical thinking, not gossip or politics. Your team will have no one to blame, and you'll feel more connected around the office. Is knowledge hoarded and privileged or is it open and shared? If you pick in pairs, there is always someone who can vouch for your actions.

Mentoring and training — by pair picking, newcomers quickly get to watch the flow of work, not just a schematic flow-chart. Instead of just an end-product, they see the clicks, the indecision, the iteration, and the pace at which tasks unfold.

Practicing pair picking is not just about sharing tasks, it is about channeling our natural social energies in the pursuit of excellence. It may not be practical all of the time, and it may make you feel vulnerable, but pairing up for seismic interpretation might bring more flow to your workflow.

If you give it a try, please let us know how it goes!

Great geophysicists #3

Today is a historic day for greatness: Rene Descartes was born exactly 415 years ago, and Isaac Newton died 284 years ago. They both contributed to our understanding of physical phenomena and the natural world and, while not exactly geophysicists, they changed how scientists think about waves in general, and light in particular.

Unweaving the rainbow

Scientists of the day recognized two types of colour. Apparent colours were those seen in prisms and rainbows, where light itself was refracted into colours. Real colours, on the other hand, were a property of bodies, disclosed by light but not produced by that light. Descartes studied refraction in raindrops and helped propagate Snell’s law in his 1637 paper, Dioptrica. His work severed this apparent–real dichotomy: all colours are apparent, and the colour of an object depends on the light you shine on it.

Newton began to work seriously with crystalline prisms around 1666. He was the first to demonstrate that white light is a scrambled superposition of wavelengths; a visual cacophony of information. Not only does a ray bend in relation to the wave speed of the material it is entering (read the post on Snellius), but Newton made one more connection. The intrinsic wave speed of the material, in turn depends on the frequency of the wave. This phenomenon is known as dispersion; different frequency components are slowed by different amounts, angling onto different paths.

What does all this mean for seismic data?

Seismic pulses, which strut and fret through the earth, reflecting and transmitting through its myriad contrasts, make for a more complicated type of prism-dispersion experiment. Compared to visible light, the effects of dispersion are subtle, negligible even, in the seismic band 2–200 Hz. However, we may measure a rock to have a wave speed of 3000 m/s at 50 Hz, and 3500 m/s at 20 kHz (logging frequencies), and 4000 m/s at 10 MHz (core laboratory frequencies). On one hand, this should be incredibly disconcerting for subsurface scientists: it keeps us from bridging the integration gap empirically. It is also a reason why geophysicists get away with haphazardly stretching and squeezing travel time measurements taken at different scales to tie wells to seismic. Is dispersion the interpreters’ fudge-factor when our multi-scale data don’t corroborate?

Chris Liner, blogging at Seismos, points out

...so much of classical seismology and wave theory is nondispersive: basic theory of P and S waves, Rayleigh waves in a half-space, geometric spreading, reflection and transmission coefficients, head waves, etc. Yet when we look at real data, strong dispersion abounds. The development of spectral decomposition has served to highlight this fact.

We should think about studying dispersion more, not just as a nuisance for what is lost (as it has been traditionally viewed), but as a colourful, scale-dependant property of the earth whose stories we seek to hear.

Great geophysicists #2: Snellius

Willebrordus Snellius (1580–1626) was the Latin name that Dutchman Willebrord Snel van Royen, or plain Snell, used for all of his publications as a mathematician. He made great advances over his predessors by introducing trigonometric methods for measuring large distances across landcapes. He's regarded as the father of triangulation, which is widely applied in surveying, imaging, and event location. And he even tried to measure the radius of the earth.

His most famous contribution, Snell's law of refraction, has formed the basis of geometrical optics and is inherently ingrained in seismology. Snell's law is used to determine the direction of wave propagation through a refractive interface:  

How much waves bend depends on the ratio of velocities between the two media v₂/ v₁. You will notice that the right hand side of this equation is where Ibn Sahl left off.

Other mathematicians before him, Ibn Sahl for instance, were aware that light rays refracted when they entered media of different velocities, but Snellius was the first to describe this problem using trigonometry. He made his discovery in 1621, when he was 41 years old, but it was never published in his lifetime. René Descartes, the inventor of the cartesian coordinate and analytical geometry, published this law of refraction 16 years after Snell's death, as Descarte's law of refraction. But Snell was eventually widely attributed with the discovery in 1703 when Christiaan Huygens published Snell's results in his Dioptrica to explain, among other things why successive wavefronts travel in parallel.

Oleg Alexandrov via Wikipedia

In a classic analogy, a 'fast' region is the beach, a ' slow' region is the water, and the fastest way for a rescuer on the beach to get to a drowning person in the water is to run, then swim, along a path that follows Snell's law. The path a ray will take upon entering a media is the one that minimzes the travel time through that media (see Fermat's principle). Notice too that there are no arrows indicating the direction of ray propagation: whether the ray enters from above or below, the refraction behaviour is the same.

In seismology, Snell's law is used to describe how seismic waves bend and turn in accordance with contrasting velocities in the subsurface which is the foundation of surveying, image focusing, and event detection. It appears in ray-tracing, ray-parameterization, offset to angle estimations (used in AVO), anisotropy problems, velocity modeling, and traveltime tomography. Snellius, we salute you!